Advanced Mathematical Research Author Md Tauseef Ibrahim/Abraham Malik: 2015-01-11

Amazon.com Widgets

http://advancedmathematicalresearch.blogspot.in/

Problem in mechanics

We are going to see a problem where we have to find the minimum or maximum value of a function.we will generate the function from Newton's Second law: The vector sum of the forces $F$ on an object is equal to the mass $m$ of that object multiplied by the acceleration vector $a$ of the object: $F = ma$ .

This can be written in the limit form as

Instantaneous acceleration is limit of the average acceleration over an infinitesimal interval of time. In the terms of calculus, instantaneous acceleration is the derivative of the velocity vector with respect to time:

\mathbf{a} = \lim_{{\Delta t}\to 0} \frac{\Delta \mathbf{v}}{\Delta t} = \frac{d\mathbf{v}}{dt}

This can be represented graphically in the vector form as shown above

The second law states that the net force on an object is equal to the rate of change (that is, the derivative) of its linear momentum p in an inertial reference frame:

\mathbf{F} = \frac{\mathrm{d}\mathbf{p}}{\mathrm{d}t} = \frac{\mathrm{d}(m\mathbf v)}{\mathrm{d}t}.

where acceleration is equal to

\mathbf{a} = \lim_{{\Delta t}\to 0} \frac{\Delta \mathbf{v}}{\Delta t} = \frac{d\mathbf{v}}{dt}

when we apply this law to any system in physics we generate a function which can be either maximized or minimized applying differential calculus or algebra.
So differential calculus come into picture.

Derivatives, Slope, Velocity, and Rate of Change.

Geometric Viewpoint on Derivatives

Figure 1: A function with secant and tangent lines

Geometrically, the limit of the secant lines is the tangent line. Therefore, the limit of the difference quotient as

h

approaches zero, if it exists, should represent the slope of the tangent line to

(a, f (a))

. This limit is defined to be the derivative of the function

f

a

f'(a)=\lim_{h\to 0}\frac{f(a+h)-f(a)}{h}.

When the limit exists,

f

is said to be differentiable at

a

. Here

f' (a)

is one of several common notations for the derivative

Let f be continuous on an interval I and differentiable on the interior of I.

If f(x)0 for all xI, then f is increasing on I.

If f(x)0 for all xI, then f is decreasing on I.

First Derivative Test
Suppose f is continuous at a critical point x0.

If f(x)>0 on an open interval extending left from x0 and f(x)<0 on an open interval extending right from x0, then f has a relative maximum at x0.
If f(x)<0 on an open interval extending left from x0 and f(x)>0 on an open interval extending right from x0, then f has a relative minimum at x0.

If f(x) has the same sign on both an open interval extending left from x0 and an open interval extending right from x0, then f does not have a relative extremum at x0.

In summary, relative extrema occur where f

(x) changes sign.

The test states: if the function f is twice differentiable at a critical point x (i.e. f'(x) = 0), then:

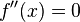If $\ f^{\prime\prime}(x) < 0$ then $\ f$ has a local maximum at $\ x$ .

If $\ f^{\prime\prime}(x) > 0$ then $\ f$ has a local minimum at $\ x$ .

If $\ f^{\prime\prime}(x) = 0$ , the test is inconclusive.

f is convex (concave-up). The slope increases from negative to positive as x increases

f is concave-down. The slope decreases from positive to negative as x increases.
Therefore, the sign of the second derivative tells us about concavity/convexity of the graph. Thus
the second derivative is good for two purposes.
1. Deciding whether a critical point is a maximum or a minimum. This is known called as the second derivative test.

The test states: if the function f is twice differentiable at a critical point x (i.e. f'(x) = 0), then:

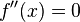If $\ f^{\prime\prime}(x) < 0$ then $\ f$ has a local maximum at $\ x$ .
If $\ f^{\prime\prime}(x) > 0$ then $\ f$ has a local minimum at $\ x$ .
If $\ f^{\prime\prime}(x) = 0$ , the test is inconclusive.

Or you can apply algebraic inequality to solve for maxima or minima problem.
using the quadratic condition.

\Delta = b^2 - 4ac.

The condition for maxima and minima depends upon delta.

All these information can well mapped to a physical problem to solve it.

Q1.A small body A starts sliding down from the top of the wedge whose base is equal to l=2.10m.The coefficient of friction between the body and the wedge surface is k=.140.At what value of the angle alpha will the time of sliding be the least?what it will be equal to?

Sol.

Consider m as the system and apply Newton's second law of motion will ease our job.

We can apply calculus to find maxima or minima where it has been done the same way.
We can also apply algebra to find the same solution.